The Pursuit of Excellence
A Manager's Guide to Quality 		Reaching New Heights


In the 1960s, Dr. Genichi Taguchi introduced a new, and more realistic loss function, to replace the traditional step function. The true nature and derivation of the Taguchi Loss function is quite complex, so it is generally given in a simplified form, as the following quadratic function:


Loss (£) = K (x - t)2
where:
x is the observed value of the characteristic X,
t is the target value, and
K is the cost coefficient

You should be happy with the definitions for x and t, but you may be wondering what we mean by the cost coefficient K.

The value of (x - t)2 will be expressed in terms of the units of measurement for X. Using the example given above, loss (x) for a sheet of thickness 13.5mm will be (13.5 - 15)2, which equals 2.25. In order to convert this into an actual monetary value, it needs to be multiplied by some constant (in this case K), which is established by calculating the known cost of some deviation from the target value.

This function may be displayed graphically as below.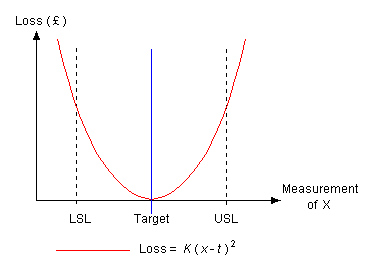

The Taguchi Loss Function